Using Game Theory to model Iran’s Nuclear Crisis
With the recent signing of the Iran Nuclear Deal arises the irrational fear that Iran will soon develop and point ICBMs at the United States. Media outlets are more than keen on exploiting this concern to stay relevant and politicians are more than keen on using this issue as a debate platform. So how likely is it that Iran will enter the nuclear age of warfare? NYU professor Bueno de Mesquita has been at the forefront of integrating game theory with political science. His probability models have correctly predicted Iran’s next Supreme Leader after Ayatollah Khomeini’s death. In 2009, he concluded that the moderates will win in the decision for nuclear armament, suggesting Iran will begin using nuclear power as an energy source but not as a bomb in the 2010s. This model works as follows:
Players: 20-30 most influential countries/parties
Strategies are devided into 2 main categories:
1. Desire for Iran to possess nuclear capabilities. Value 0-200 (0=strongly against, 100=neutral, 200=strongly agree)
2. Amount of influence each country has on the outcome. Value 0-100 (little-great influence)
Goal of every player: Have overall likeliness of Iran possessing a nuclear weapon (a value 0-200) come as close to its own desire value as possible. The overall likeliness of Iran possessing a warhead is calculated using a weighted average: summation of each player’s(influence*desire)/(sum of each player’s influence).
Payout for every player: |Overall likeliness-Desire|, minimise this value.
He concluded Iran’s likeliness of nuclear armament as a value of 118. These calculations take highly trained computers hours to compute. For the sake of simplicity, we assign each such that the new game definition becomes.
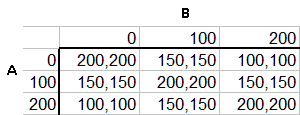
Players: Country A, Country B
Strategies: 0=against, 100=neutral, 200=agree
Payout for each player: 200-|Overall likeliness-Desire|, each player wishes to maximize this payout.
This generates the attached payoff matrix. The Pure Nash Equilibria for this game would be {(0,0),(100,100),(200,200)}. However in political context, each country or faction would be aware of extremist opponents and would receive a more generous payoff with the more moderate strategy: (1,1).
Main article: http://www.nytimes.com/2009/08/16/magazine/16Bruce-t.html?pagewanted=all
For context about the Iran Nuclear Deal: http://www.npr.org/sections/thetwo-way/2015/07/14/422920192/6-things-you-should-know-about-the-iran-nuclear-deal
