The North Korean Missile Crisis and What Game Theory Teaches Us
I have recently read Cornell University Professor Kaushik Basu’s article within Project Syndicate titled “The North Korean Missile Crisis” and within it Basu compares the current North Korean situation to the Cuban Missile Crisis. His claim is that the current status quo, in which the world is seeing nuclear instability from a feared nation-state has only one precedent in modern history: the Soviet Union’s placement of ballistic missiles in Cuba in 1962. Basu suggests that the reason that the Cuban Missile Crisis did not end in complete failure and nuclear destruction is that both sides applied analytical game theory to maximize their payoffs. It is now publicly known that John F. Kennedy seeked the advice of the Nobel Prize winning economist and renowned game theorist Thomas Shelling and it is his game theory approach that helped deescalate the Cuban Missile Crisis. However, what Basu does note is that the North Korean problem has a different model from the Cuban Missile Crisis and the same strategy cannot be applied and the Hawk-Dove game simplification is inapplicable. North Korea, by virtue of its geographic location, is a buffer for China. If the United States were to overtake North Korea, it would pose a security threat to China. And so, what started as a two-player game, as Basu notes, has become a three player game. The game has changed and the outcome now is not as determined.
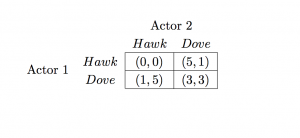
Although we may not be able to solve the North Korean Nuclear Crisis using our Networks knowledge, it is relatively simple to use our Networks knowledge to understand the game theory approach that Kennedy sought in the Cuban Missile Crisis. As Basu suggests, many international relations problems including the Cuban Missile Crisis can be modeled by a game introduced in class: the famous Hawk-Dove game. The payoff matrix for the game is as follows:
The Nash Equilibrium for this game is (Dove, Hawk) and (Hawk, Dove) with no dominant strategy. And so, if we play this game like the Prisoner’s Dilemma, either of those two outcomes should eventually happen. However, in international relations, countries and actors do not necessarily move simultaneously. There is very often an aggressor or first move actor and in the case of Hawk-Dove, game theory teaches us that it will always benefit you to make the first move and play Hawk. The reason for this is that if the other actor is rational, it will always choose Dove to gain some payoff and so (5,1) will be the outcome with the aggressor getting the maximum payoff. And so this explains Kennedy’s action perfectly in that he was aggressive in the crisis and quickly announced a naval blockade, which shortly ended the crisis.
Unfortunately, we cannot model the North Korean crisis in the same way as the Hawk-Dove game is only applicable for two actors. A question that will come up with three actors is how to define the payoff for (Hawk, Hawk, Dove), in which two of the actors, let’s say the United States and China, are aggressive and a third, North Korea, is not. And if such a payoff is defined, will it even benefit an actor to be a first aggressor? Another question that will come up is even if there is a strategy for the United States, can we even model it using game theory under the assumption that Kim Jong Un and North Korea are rational actors?
Source: https://www.project-syndicate.org/commentary/north-korea-nuclear-threat-game-theory-by-kaushik-basu-2017-07