A Game Theory Analysis of Doklam Standoff
Recently, India, China and Bhutan are engaged in a standoff in the tri-junction area of the boundary of the three states in Doklam. It is believed that the current standoff could well turn into a military confrontation as both India and China have refused to budge. China has insisted that India pulling back from Chinese territory is a precondition for any talks, while Indians has demonstrated its determination on mutual withdrawal. Obviously, the topic of major debate for either of the two countries is about whether to resolve the issue through collaboration or maintain the existing positions.
In order to construct a Game Theory model of the Doklam standoff, the writer assumes that China and India are the two players in the game that have to choose between two strategies: collaboration or collusion. The collaboration strategy means to withdraw and seek for resolving the issue through bilateral or trilateral talks, and the collusion strategy means to maintain the existing positions on the Boundary Dispute.
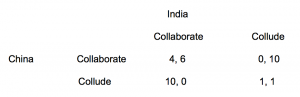
When China and India both choose to collaborate, potential armed confrontation would be avoided. Also, an environment of confidence would be created, along with a greater mutual economic benefit. However, China is at a more significant disadvantage to choose to collaborate because it would show signs of weakness, imply losing a strategic gambit to improve defensive positions astride astride the Chumbi Valley, and lose face for the leadership. In this case, we can assume that the payoff of China is 4 and that of India is 6.
If China choose to collude and India choose to collaborate, China would be able to extend its road on the Doklam plateau while India would lose the control of the disputed area. In this case, China’s payoff is 10 and India’s payoff is 0. On the contrary, if China choose to collaborate and India choose to collude, India will benefit from this strategy and get a payoff of 10, while China’s payoff is 0.
Lastly, if China and India both choose to collude, a military confrontation may become inevitable. In this case, the payoffs of both China and India are assumed to be 1.
We can thus establish the following payoff matrix:
It is clear from the payoff matrix that Collaborate becomes a strategy that neither China nor India should use as there is another strategy that always yields a higher payoff (Collude). Thus, the only Nash equilibria here is for both countries to collude, even though (Collaborate, Collaborate) actually yields a significantly higher payoff for both countries. This analysis matches the conclusion given in the article, that continued standoff seems imminent, and India will have to convince China that it is beneficial for both sides to break the deadlock while preparing for an armed conflict in the future.
Article Links:
http://www.business-standard.com/article/economy-policy/india-china-standoff-does-it-make-sense-for-india-to-mount-barricades-at-doklam-117080200199_1.html
https://www.linkedin.com/pulse/india-china-doklam-game-theory-rahul-bhonsle