Applying Power Laws to Stock Markets
For at least 100 years, academics have applied physics to economics and the financial markets. The field, sometimes called econophysics, was first pioneered by Mandelbrot and Pareto. Using cotton prices, Mandelbrot found that the distributions of returns for different time intervals could be modeled using the same exponent. That is, returns measured by the month experienced an exponent similar to returns measured by the day. More recently, Gopikrishnan (1999) found a similar result in various global stock markets. He sought to model the distribution of stock market returns. His research initially began with a database that contained many millions of data points, each recorded at a frequency of 15 seconds. To measure S&P 500 return frequencies of one minute, a sample was taken from this database of 15 second return frequencies. This same process was utilized up until daily and monthly frequencies were desired, at which point separate databases of different years were used. Gopikrishnan found power law P behavior of normalized returns g of
P(g > x) ~ 1/x^a
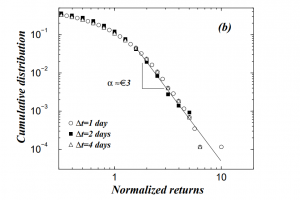
that is nearly identical to the form used in class. Regression fitting was then used to find a. For all frequencies of return records, an exponent of 3 was found. Not all returns were analyzed. When analyzing daily returns frequencies, only normalized returns between 1 and 10 were fit with regression. The following graph was taken directly from Gopikrishnan (1999). The x-axis depicts the normalized returns while the y-axis depicts distribution. The power of 3 is noted in the center. Returns measured at frequencies of 1, 2, and 4 days are shown.
Again, this same inverse cubic power law was evident not only across frequencies of 1, 2, and 3 days, but also at the minute and monthly levels. To check for United States specific phenomena, the Japanese NIKKEI and the Hang-Seng were also analyzed. These markets experienced the same inverse cubic law. For those interested in more applications of power laws in economics and finance, Power Laws in Economics and Finance is a fine literature review on the subject.