The game theory behind Badminton service
This research paper discusses strategy in singles Badminton service. It discusses two key strategies. Players can either use a high-risk serve (a high serve to the back of the court) or a low-risk serve (a short serve to the front). The high risk serve is more risky because if the receiver anticipates a high serve to the back, they can return it aggressively. However, if the receiver is caught off-guard by a high or low serve, they are at a disadvantage. This paper uses game theory analysis to determine how frequently high and low risk serves should be used and how frequently opponents should anticipate high and low risk serves.
I have played Badminton for 10 years and have always arbitrarily decided between high and low serves. This may not be the optimal strategy, however, as there may be certain patterns which I follow subconsciously, such as using high-risk serves after tiring rallies. This could allow opponents to anticipate my serves, giving them an advantage. Instead, through the use of game theory we may be able to determine a method to maximize the chance of winning.
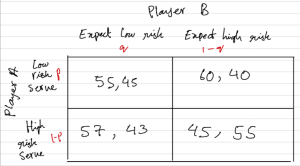
We can model the different options using a table where p is the probability that Player A uses a low-risk serve and 1-p is the probability they use a high risk serve. Similarly, q is the probability that Player B expects a low-risk serve and 1-q is the probability that player b expects a high-risk serve. The pay-off values in the table were based on the values provided by the article.
There is clearly no dominant strategy given that no single strategy creates an optimal outcome for either player. Furthermore, there is no pure Nash equilibrium because players can gain by changing their strategy when their opponent’s strategy remains unchanged. We can, however, calculate mixed strategies which determine probabilities for p and q which will form a mixed nash equilibrium.
This makes it clear that the optimal strategy for either player is to randomize their service style and follow roughly the calculated values. This creates a system of mutual best responses for both players. In order to make this method truly useful, players should populate the table with pay-off values from previous encounters with an opponent and then use those values to create a mixed strategy. For example, if in previous encounters, Player A won 3 quarters of rallies when they completed a high-risk serve and player B anticipated a low-risk serve, the pay-off value in the bottom left corner would be 75, 25.