Polygons, Segregation, and Nash Equilibrium
While researching ideas for my blog post I came across a website Parable of the Polygon created by Vi Hart and Nicky Case. This website creates an interactable model demonstrating the Thomas Schelling 1971 paper Dynamic Models of Segregation. Schelling’s segregation model essentially states that in a larger system –or game –of housing/neighborly relationships small individual biases can domino effect into a large collect bias (i.e. segregation).
In Parable of the Polygon, this game of neighbor dynamics is represented by squares and triangles that want to have at least a certain percentage of their neighbors to be their own shape. If triangles/squares’ needs are not met (and are thusly unhappy) the website challenges us to relocate each unhappy polygon. At a preference for greater than or equal to 33%, similar neighbors the effect of the unsatisfied individual segregating the community becomes jarringly apparent (see figure 1 and figure 2).
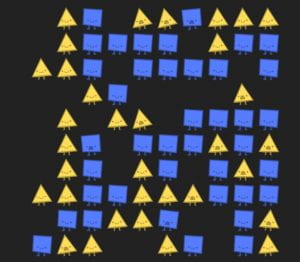
FIG 1: 33% neighbor preference before sorting (unsatisfied people-characterized by shapes with gaping mouths).
FIG 2: 33% neighbor homogeneity after sorting (satisfied, but now the neighborhood is segregated).
Parable of the Polygons allows us to simulate this phenomenon with other percentage values through running simulations. It turns out that any percentage above 33% consistently yields a dramatically segregated neighborhood. These staggering results seem to mirror the racial politics regarding the long battle for desegregation in the United States. In fact, desegregation is still being fought today. During as recent as 2016 NPR reported on a Missippi school district finally receiving an order to desegregate.
I believe that the forces at work behind Schelling’s segregation model is very similar to the mixed/pure Nash Equilibrium traffic games that we encountered in class. Instead of drivers, we have polygons, and in the place of roads, we have the unoccupied spaces. In other words, in this game, each unhappy polygon is a player that will need to pick a “strategy” (i.e. find new empty space to move to) based on the “payoff” that the new space provides (i.e. payoff = compliance with the percentage condition). Just like our drivers, the polygons will eventually reach a NE (though the NE may likely be segregated).
So–what are some solutions to that may reverse this segregated result? Simply lowering the bias yields no change in the segregated system since technically living with at least 55% similar neighbors does not violate the lowered condition that one must live with at least 22% similar neighbors.
The solution to this problem is to demand diversity. In other words having a range of preferred similar neighbors, rather than a strict value. Thus from my experience using Parable of the Polygon I learned that in a segregated neighborhood easily influenced by individual bias, the solution is to demand diversity.
Sources:
https://ncase.me/polygons/
https://www.npr.org/sections/thetwo-way/2016/05/17/478389720/after-50-year-legal-struggle-mississippi-school-district-ordered-to-desegregate