Saving Africa’s Elephants with Game Theory
Currently many species of elephants are endangered and facing extinction in Africa. With ivory always in high demand, even with current international regulations against poaching, elephant poaching is still a major issue. To help fight this, in 2016, the Chinese government announced a domestic ivory trade ban, ending the legal domestic ivory markets in China. Even though international regulations were in place, the Chinese legal markets allowed poachers a method to launder their illegal ivory. Although the new ban had immediate results in terms of market price, many people still believed the ban was useless due to underground deals that were still surely occurring.
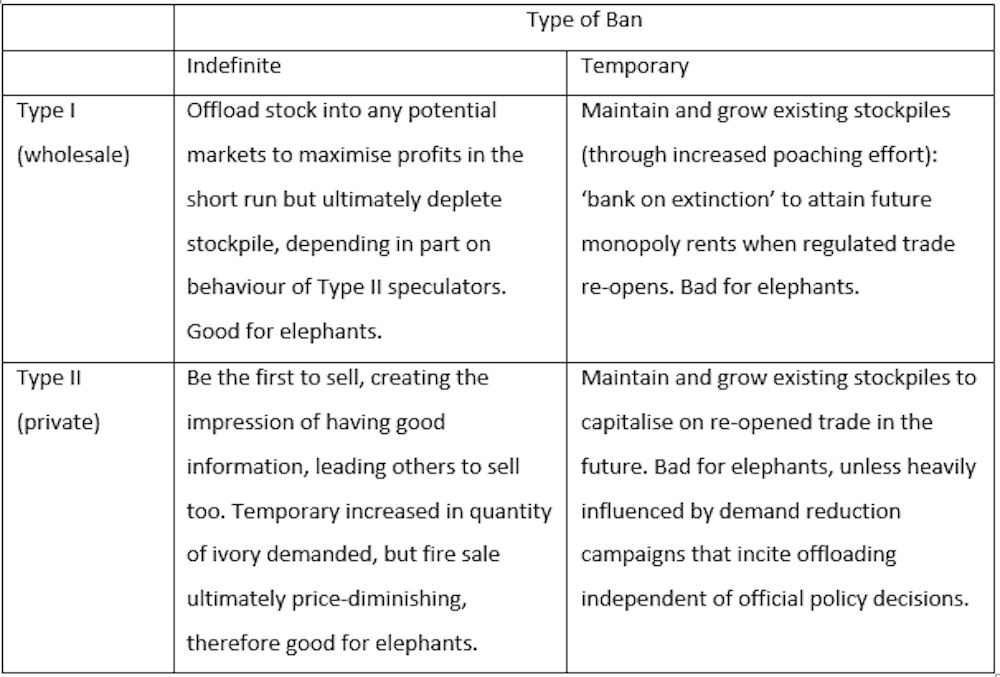
The author of this articles uses game theory to predict how poaching likely fairs due to different policies and whether or not these enacted policies should be permanent or only temporary. Weighing the payoffs for two different types of poachers, wholesale and private, the author creates a payoff matrix for both these types of poachers and the policy makers.
For indefinite bans, the author predicts that the poachers would most likely dump their ivory stock as quickly as possible to liquidate their product at the highest price before it inevitably drops. The mass panic among private ivory poachers to sell all their stock in case the price drops would force wholesale poachers to offload their own stock actually causing the market price to drop. A price drop in ivory would benefit elephants as poachers would be less likely to risk their lives to illegally poach elephants if the reward was less. On the contrary, the author predicts that if the ban is temporary, poachers are more likely to hold onto their stock until the ban ends and would continue poaching elephants to build their stock up after the ban ends.
With player A representing the poachers and player B representing the policy makers/regulators, the author creates a payoff matrix to show the various payoffs for each party. If B sets the ban to be indefinite, player A would liquidate their stock for the higher payoff instead of waiting for elephant extinction. On the other hand, if B sets the ban to be temporary, not only would they be losing out on payoffs for themselves, but also player A would simply bank on elephant extinction and continue poaching. Based on this matrix, clearly the dominant strategy for policy makers is to set the ban to be indefinite to best save elephant lives.
This article demonstrates how game theory and payoff matrices can be used in real world applications. We can split poachers and policy makers into players in this “game.” Based on work in class, we can determine the dominant strategy for player B, the policy makers, and the Nash equilibrium for both sides at the bottom left position of the payoff matrix.
https://theconversation.com/game-theory-suggests-china-should-keep-its-ivory-trade-ban-in-place-indefinitely-78613