The Role of Competition and Cooperation in Evolutionary Game Theory
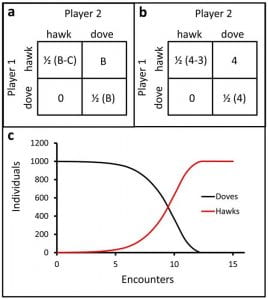
When analyzing the role Game Theory plays in nature, one might be led to believe that the dominant theory is always to follow the “survival of the fittest” individualistic strategy of putting one’s own needs above others. But in this article, we see through the lens of game theory that those who employ a mixture of cooperation and altruism among these interactions alongside the usual aggression result in the most evolutionary stable strategies in the long run. These evolutionarily stable strategies are defined as those that cannot be replaced or invaded by any other strategy through natural selection. However, because evolutionary game theory deals with aggregate populations in the long run moreso than individual experiences, the resulting evolutionary stable strategies are generally seen through the long-run pervasive traits of a population. For instance, in the case of the relationship between strategies employed by doves and hawks, passive and aggressive, we observe that the aggressive strategy of the hawk can only be evolutionarily advantageous in the long run if the benefit of that aggressiveness in the environment outweighs the cost of competition with similarly matched predators. If a clear size advantage exists, it is often that the less well suited of the pair flees; however, when those of similar abilities encounter one another and the advantage is often unclear, the payoff for both individuals becomes blurred leading to a clash in which both entities incur a cost surpassing the possible benefits of cooperation. As demonstrated in the provided matrix below, the greatest benefit to cost ratio arises when both members employ a dove strategy; however, in the long run this cannot become a evolutionarily stable strategy due to the nature of the relationship between the two species. Any low number of hawks would over time expand territories diminishing the dove population, creating exponential growth until the cost of conflict becomes greater than the reward, then neither the hawk nor the dove strategy satisfies the conditions of ESS as a pure strategy.
The “game” being employed within nature does deviate from what we have learned in class thus far in that 1) our “players” cannot be assumed to be rational thinkers in the exact same way that people are and 2) the nature of evolutionary game theory is that of entire populations rather than two or more individuals in a single encounter. As such, we encounter game theory from a perspective of population wide learning experiences, one in which subsequent iterations of these games are influenced by the successful or unsuccessful experiences of previous games. In class we discussed in depth the iterations of the Prisoners Dilemma, a game in which we solely viewed a single decision; the large caveat, and one in which we will discuss further in depth later in the semester, is that this game must take into account the likelihood that one player’s decision to cooperate in one game, such as a bird species calling out loudly to warn others of its species of predators and therefore putting itself in danger, would likely influence the rest of the population to employ this strategy in the future. Though in the short run this might lead to certain individuals dying off from predators, the long run iterations of the game in this network must eventually produce the most successful evolutionary strategies.